Journée Algèbre Linéaire à Amiens

lundi 17 mars 2025, 09:30 → 17:00 Europe/Paris

Sophie Germain (Amiens - UFR des Sciences)

Présentation de la journée

Cette journée a pour but de rassembler les acteurs mathématiques de la région Hauts-de-France autour de l'algèbre linéaire numérique et de ses applications.

Orateurs et oratrices

• Yousef Saad (Université du Minnesota)
• Marcos Raydan (Université Nova de Lisboa)
• Antoine Levitt (Université Paris-Saclay)
• Nicole Spillane (CNRS & École Polytechnique)
• Youcef Mammeri (Université Jean Monnet)

Inscriptions

Inscription gratuite mais obligatoire. Le nombre de participant⋅e⋅s est limité à 30 personnes.

Lieu de la journée

La journée se déroulera à l'UFR des Sciences, 33 rue Saint-Leu, 80039 Amiens, en salle Sophie Germain.

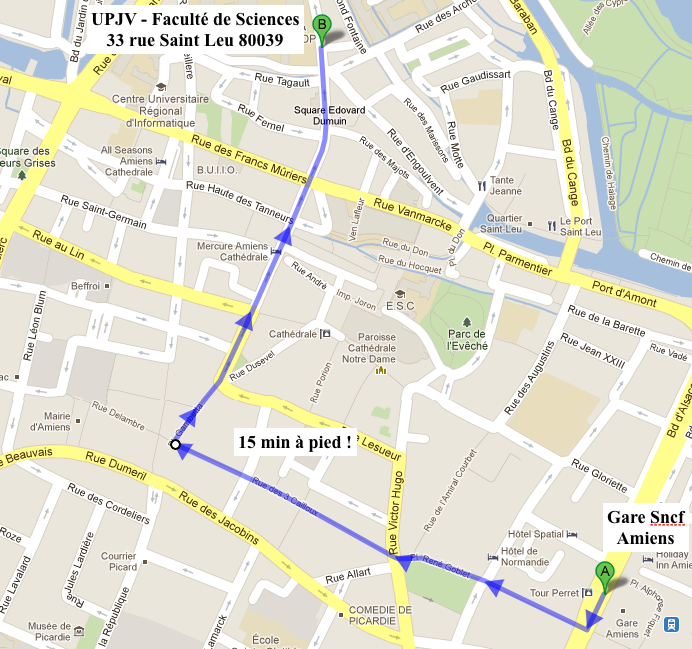
Vous trouverez un plan pour venir au 33 rue Saint-Leu depuis la gare ici.

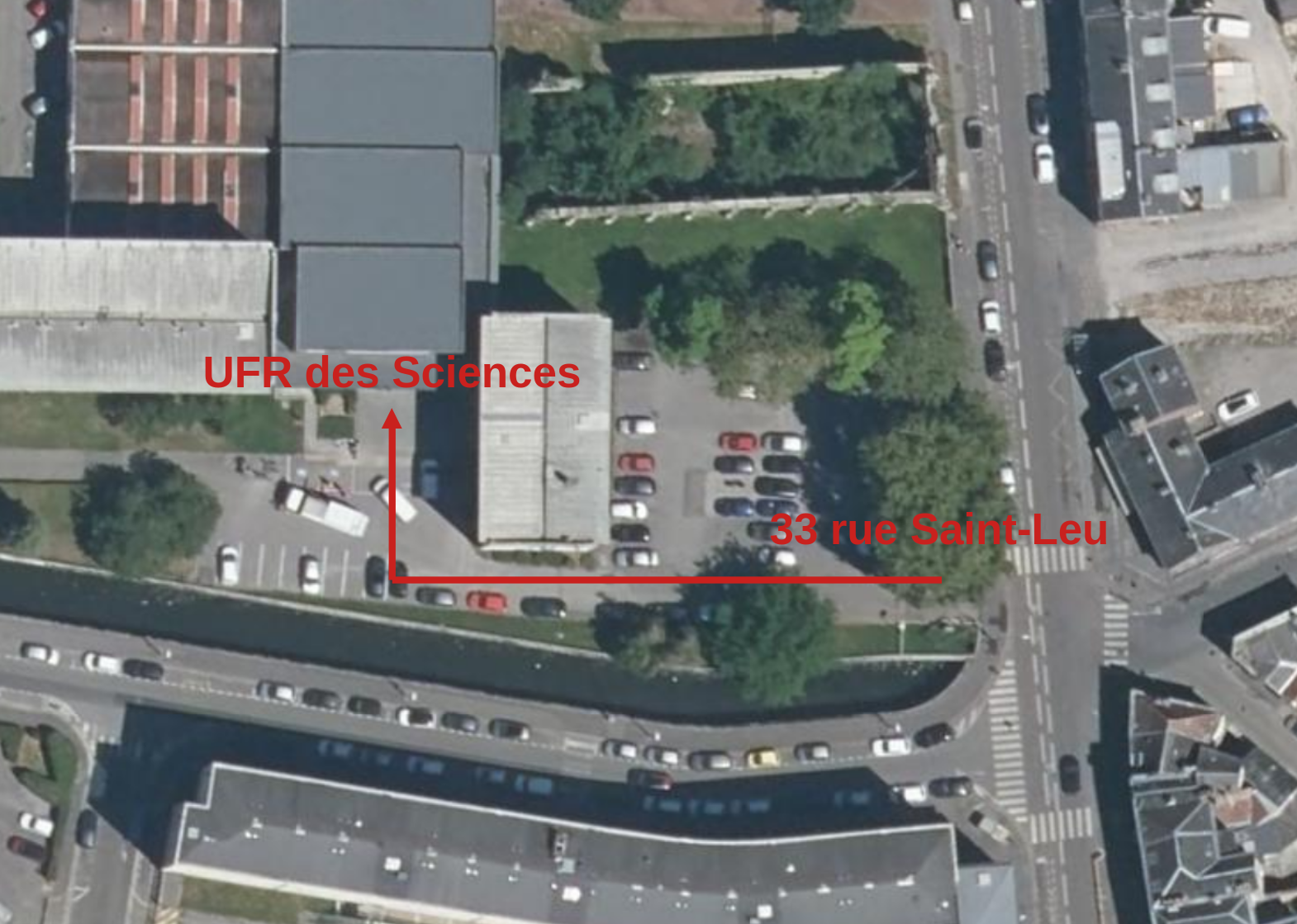
Une fois sur place, l'UFR se trouve derrière le parking, et la salle Sophie Germain se situe au fond du hall d'entrée sur la droite.

Financements

Cette journée est financée par le LAMFA et l'Université de Picardie Jules Verne.

 

09:30 Accueil

1 A class of nonlinear acceleration techniques based on Krylov subspaces¶

There has been a surge of interest in recent years in general-purpose
acceleration' methods that take a sequence of vectors converging to the limit of a fixed point iteration, and produce from it a faster converging sequence. A prototype of these methods that attracted much attention recently is the Anderson Acceleration (AA) procedure. We introduce the nonlinear Truncated Generalized Conjugate Residual (nlTGCR) algorithm, an alternative to AA which is designed from a careful adaptation of the Conjugate Residual method for solving linear systems of equations to the nonlinear context. The various links between nlTGCR and inexact Newton, quasi-Newton, and multisecant methods are exploited to build a method that has strong global convergence properties and that can also exploit symmetry when applicable. Taking this algorithm as a starting point we explore a number of other acceleration procedures including a short-term (symmetric') version of Anderson Acceleration which we call Anderson
Acceleration with Truncated Gram-Schmidt.

Orateur: Yousef Saad

2 Convergence of GMRES accelerated by deflation and preconditioning¶

This is joint work with Daniel Szyld (Temple University)

We present new convergence bounds for weighted, preconditioned, and deflated GMRES applied to non-Hermitian linear systems. These bounds are given for the case when the Hermitian part of the coefficient matrix is positive definite, the preconditioner is Hermitian positive definite, and the weight is equal to the preconditioner. The decrease in residual is bounded with respect to:
- the condition number of the preconditioned Hermitian part of the problem matrix,
- certain measure of how non-Hermitian the problem is. This indicates how to choose the preconditioner and the deflation space in order to accelerate convergence. One such choice of deflation space is proposed, and numerical experiments illustrate the effectiveness of such space.

Orateur: Nicole Spillane

11:50 Pause repas

3 Perturbations of functions of matrices and applications to density functional theory¶

Orateur: Antoine Levitt

4 Enhanced stabilization strategies for some linearized PDEs¶

Stabilizing linearized partial differential equations (PDEs) is a fundamental challenge in numerical analysis and control theory. In this talk, we present advanced strategies for enhancing the stability of linearized PDEs. We begin by reminding the concept of linear instability, illustrated through the example of the Klein-Gordon equation. Next, we introduce an optimal stabilization approach, based on the minimization of the Frobenius norm of the discrete linearized operator and its associated second-order eigenvalue problem. Building on this, we explore automatic stabilization using PyTorch, demonstrating how machine learning techniques can adaptively stabilize PDEs. We showcase the effectiveness of these methods through a case study, of the Klein-Gordon and the Korteweg-de Vries equation. Finally, we discuss broader implications, including stabilization at every time step and connections to approximate controllability. This is a joint work with J.P. Chehab and M. Raydan.

Orateur: Youcef Mammeri

15:40 Pause goûter

5 Continuous optimization strategies for inverse structured matrix problems with prescribed spectrum¶

The talk will consider several continuous optimization schemes to solve inverse structured symmetric matrix problems with prescribed spectrum. Some entries in the desired matrix are assigned in advance and cannot be altered, and some others should be nonzero. The rest of the entries are free. The reconstructed matrix must satisfy these requirements and its eigenvalues must be the given ones. This inverse eigenvalue problem, that appears in several applications, is related to the problem of determining the graph with weights on the undirected edges, of the matrix associated with its sparse pattern. Our optimization schemes are based on considering the eigenvector matrix as the only unknown and moving iteratively on the manifold of orthogonal matrices, forcing the additional structural requirements through a change of variables and a convenient differentiable objective function in the space of square matrices. We propose and analyze Riemannian gradient-type methods combined with either a penalization or an augmented Lagrangian strategy. We also present a block alternating technique that takes advantage of a proper separation of variables. We present some numerical results to demonstrate the effectiveness of our proposals.

Note:
This is a joint work with Jean-Paul Chehab (Amiens, France) and Harry Oviedo (Chile).

Orateur: Marcos Raydan